Simulations for the paper

"Packungen aus Kreisscheiben"

Charlotte Dombrowsky, Myriam Fradon, Sylvie Roelly

Illustration of the convergence towards equilibrium states

Numerical simulation of the hard sphere stochastic dynamics with attractive interaction.
The number on the left is the mean energy (second moment).
Hard spheres with radius 1. Size 10 for the background squares. At time zero, the ball locations are random. The balls move according to a Brownian motion, they interact through a pair attraction with coefficient a and elastic collisions.
Position computation with Scilab. Sphere visualisation with PoV-Ray. Compression of the pictures using mp4 encoding.

Convergence towards a configuration on the triangular lattice

Simulation of 250 spheres, attraction a=0.005

Simulation of 250 spheres, attraction a=0.0025
The attraction coefficient is smaller than above, hence the lesser density of the equilibrium state.

Simulation of 50 spheres, attraction a=0.05

Simulation of 500 spheres, attraction a=0.0005
The computation time increases as the square of the number of balls. It is roughtly proportionnal to the number of collisions (event-driven algorithm). The heavy computation for 500 balls becomes slower the closer we get from the minimum energy (incresing number of collisions). This computation was interrupted after 60 hours..

Hexagonal clusters

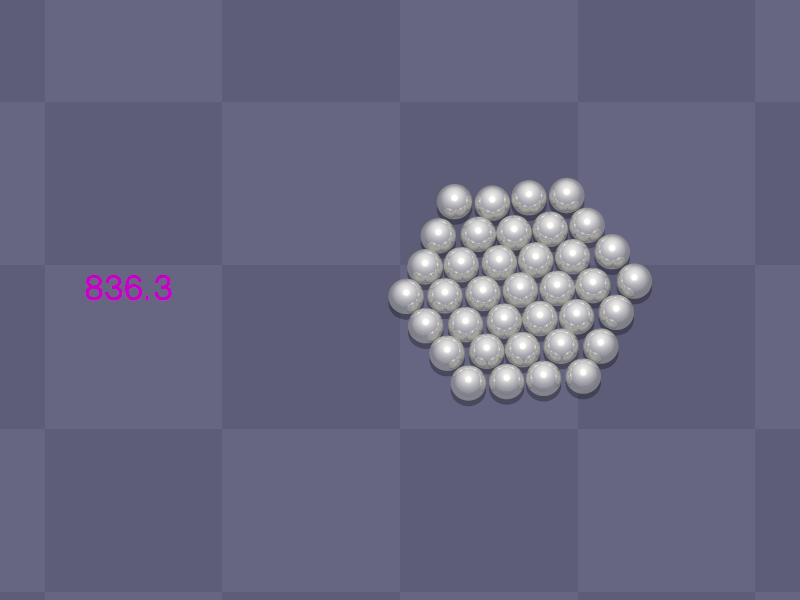
Simulation of 19 spheres, attraction a=1
19 is the third hexagonal number. The mean energy of the hexagonal configuration is 192.
The motion of the almost hexagonal sphere cluster is Brownian, √19 times slower than the individual Brownian motions of the spheres.

Simulation of 37 spheres, attraction a=1
37 is the fourth hexagonal number. The mean energy of the hexagonal configuration is 744.
Fast convergence towards a lattice configuration, but it takes a long time to reach a quasi-hexagonal configuration. The hexagonal cluster in the last part of the simulation is Brownian and √37 times slower than the spheres.
Warning : slow convergence hence long movie (1h20, 1,4Go)

Simulation of 38 spheres, attraction a=0.1
The dynamics explores several lattice circular configurations, ending with the additionnal ball aside a quasi-hexagon.
Warning : slow convergence hence long movie (1h20, 1,4Go)

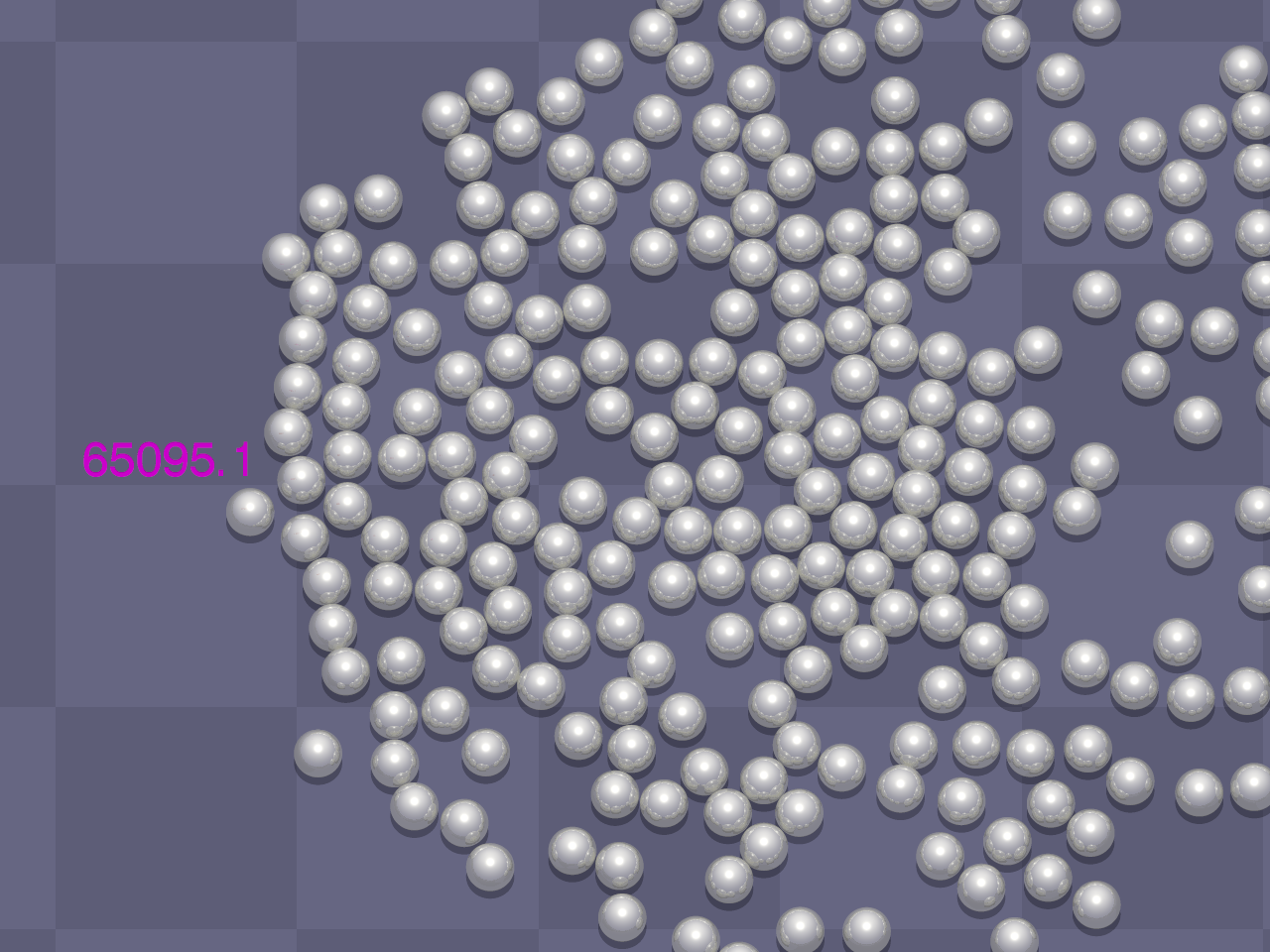
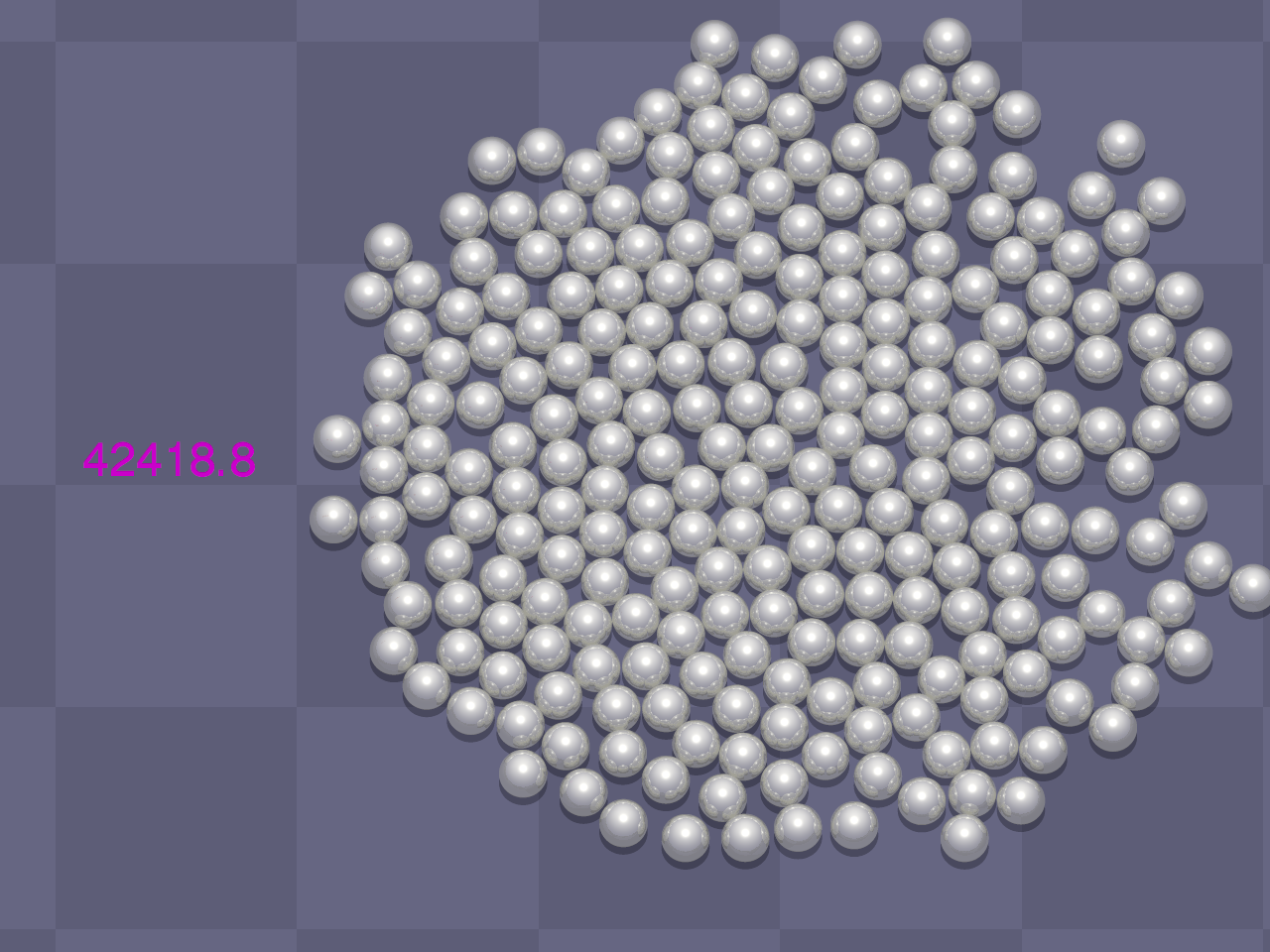
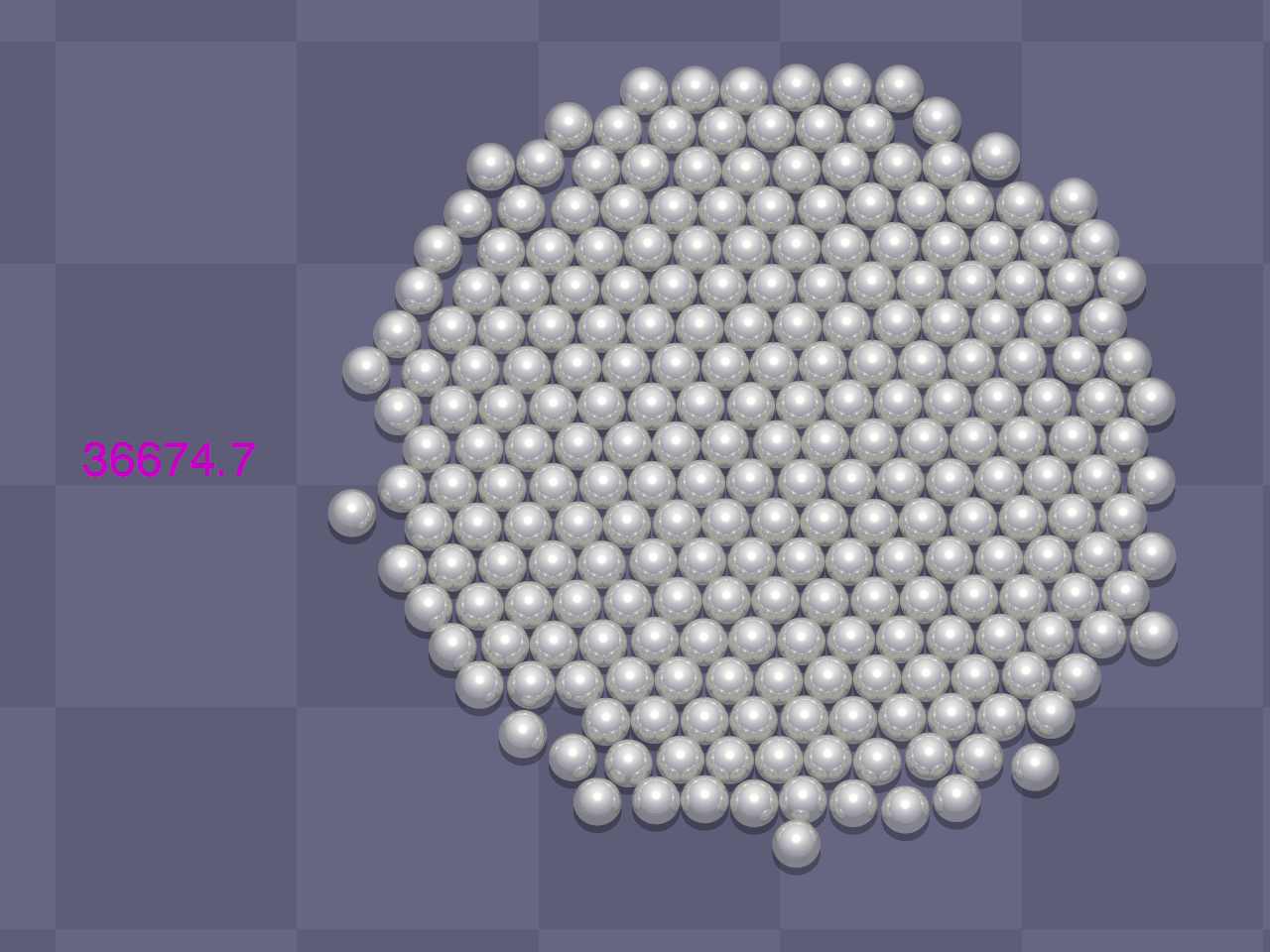
Closeup of the dynamics for 250 spheres

Back to the main page